Use the divergence test to determine whether a series converges or diverges Use the integral test to determine the convergence of a series Estimate the value of a series by finding bounds on its remainder term In the previous section, we determined the convergence or divergence of several series by explicitly calculating the limit of the Show Solution To determine if the series is convergent we first need to get our hands on a formula for the general term in the sequence of partial sums s n = n ∑ i = 1 i s n = ∑ i = 1 n i This is a known series and its value can be shown to be, s n = n ∑ i = 1 i = n ( n 1) 2 s n = ∑ i = 1 n i = n ( n 1) 2 Sequences Convergence and Divergence In Section 21, we consider (infinite) sequences, limits of sequences, and bounded and monotonic sequences of real numbers In addition to certain basic properties of convergent sequences, we also study divergent sequences and in particular, sequences that tend to positive or negative infinity We
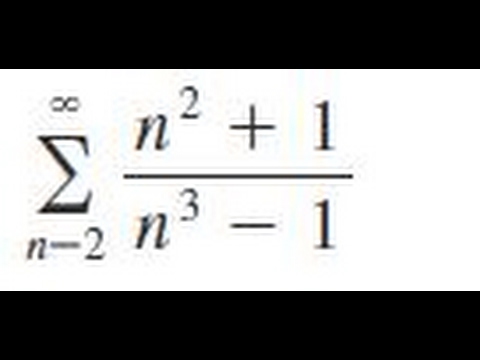
Sigma 2 Infinity N 2 1 N 3 1 Determine Whether The Series Converges Or Diverges Youtube
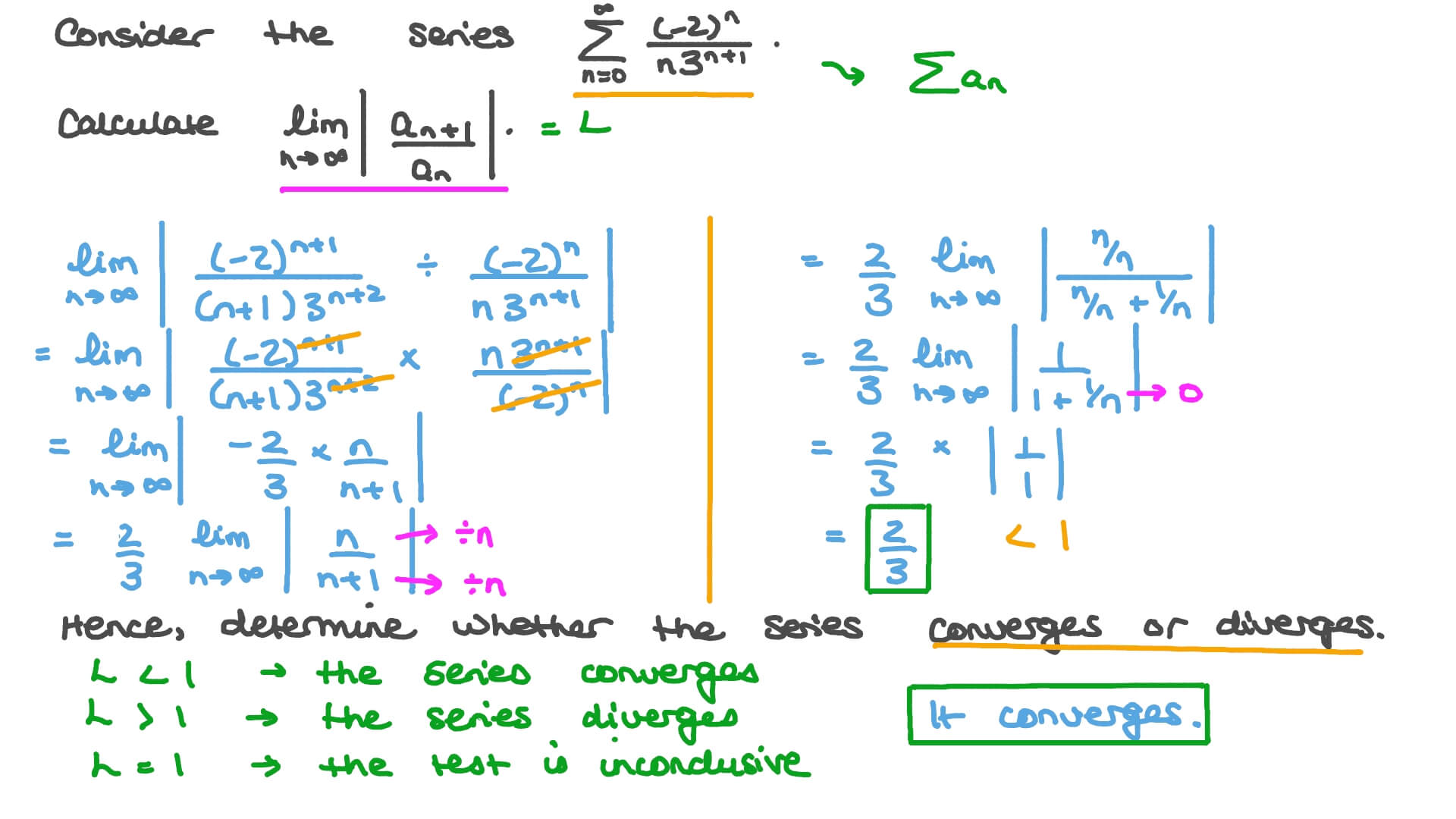
Does (2/3)^n converge or diverge
Does (2/3)^n converge or diverge-Diverges, LCT with P 1 n which diverges, or directly compare 2n1 3n24 2n 3 2 = 1 2n and 1 2 P 1 n diverges (f) X 1 ( 1)n n diverges, Test for Divergence 4Determine if the following series absolutely converge, conditionally converge, or diverge (a) X sinn n2 1 converges absolutely, direct comparison 0 jsinn n21 j 1 and P 12Determine if the following series converge or diverge You must show all of your work and justify your use of any series convergence tests (a)(7 points) X1 n=1 n3 3n Solution Consider the ratio test lim n!1 (n 1)3 3n1 3n n3 = lim n!1 (n 1)3 n3 3n 3n1 = 1 1 3 = 1 3 Since this limit L




Calculus Sum Of 1 To Infinity Of Ln N Sqrt N Convergent Or Divergent Learnmath
The sequence diverges 3 X∞ n=2 n2 1 n3 −1 The terms of the sum go to zero, since there is an n2 in the numerator, and n3 in the denominator In fact, it looks like P 1 n, so we compare it to that lim n→∞ n2−1 n3−1 1 n = lim n→∞ n3 −n n3 −1 = 1 Therefore, the series diverges by the limit comparison test, with P 1 n 4 X∞ n=1 5−2 √ n n3531 Use the divergence test to determine whether a series converges or diverges 532 Use the integral test to determine the convergence of a series 533 Estimate the value of a series by finding bounds on its remainder term In the previous section, we determined the convergence or divergence of several series by explicitly calculatingConverge or both diverge lim 0n n n a c c →∞ b n=2 n=3 n=4 n=5 n=6 n=7 n=8 Is the alternating sequence converging?
Give reasons for your answers (When you check an answer, remember that there may be more than one way to determine the series' convergence or divergence) $$ \sum_{n=1}^{\infty} \frac{2^{n}}{3^{n}} $$ In order to converge, the limit of the terms of the sequence must approach 0;Let a n = 2 n 3 n 1 (a) Determine whether {a n} is convergent (b) Is ∞ X n =1 a n is convergent?
Ask Question Asked 8 years, 3 months ago Active 8 years, 3 months ago Viewed 43k times 13 7 $\begingroup$ so I used the root test, but i'm not quite sure if i'm allowed to I think im performing the operations correctly,a and i keep ending up with $(1)^{\infty}$Thus the series on the left diverges as well, and adding back in the finite number of terms for n < N does not affect the result Thus ∞ n = 2 1 (ln n) 4 diverges 62 ∞ n = 1 2 n 3 n − n solution Apply the Limit Comparison Test with a n = 2 n 3 n − n and b n = 2 n 3 n L = lim n →∞ a n b n = lim n →∞ 2 n 3 n − n 2 n 3 n = limFor convergence or divergence Solution We have a n = 2n 3n 1 n→∞ a n b n = c, and if 0 < c < ∞, then either both series converge or both diverge The limit comparison test follows from the basic comparison test Indeed, we may choose two real



What Is The Radius Of Convergence On The Power Series From 0 To The Infinity Of 1 N 3 N 2 N X N Quora



Geometric Series Test To Figure Out Convergence Krista King Math Online Math Tutor
Explain your answer X1 n=1 n 3n Answer Use the Ratio Test lim n!1 n1 3n1 n 3n = lim n!1 n 1 3n1 3n n = lim n!1 1 3 n 1 n = 1 3 Since 1 3Converge or Diverge?—Comparison and Limit Comparison Learning goal Students start to use more convergence tests—comparison and limit comparison tests are introduced, and pseries are used as the basis for many comparisons For what values of p does the series 1 np n=1 ∞ ∑ converge? Does the series converge or diverge?



Answered Does The Sequence An Converge Or Bartleby



Http Dept Math Lsa Umich Edu Zieve 116 Series2 Solutions Pdf
Lim n → ∞ (2 n 1) / 3 n 2 n / 3 n = 1 lim n → ∞ (2 n 1) / 3 n 2 n / 3 n = 1 Since ∑ n = 1 ∞ ( 2 3 ) n ∑ n = 1 ∞ ( 2 3 ) n converges, we conclude that ∑ n = 1 ∞ 2 As you correctly note, this is e 3 − 1 That's because the series for e x converges everywhere!So you are done, it converges (specifically to e 3 − 1 ) When saying that you have 'reduced it' to e 3 − 1 which none the less is correct and thus the series converges to that particular value you most likely used that e x = ∑ n x n / n




Calculus Sum Of 1 To Infinity Of Ln N Sqrt N Convergent Or Divergent Learnmath



Will Series N N 1 Converge Or Diverge As N Tends To Infinity Quora
To converge Similarly, a given improper integral diverges if its integrand is greater than the integrand of another integral known to diverge We now apply the same idea to infinite series instead Direct Comparison Test for Series If 0 ≤ a n ≤ b n for all n ≥ N, for some N, then, 1 If X∞ n=1 b n converges, then so does X∞ n=1 a nAnswer to Does this series converge or diverge?This sum divergeswe can say this by using the pseries theorem eg we have a series summation (n^p) where n goes from 1 to infinity then if p>1 then the series converges and if p



Sigma 1 Infinity 1 Sqrt N 3 1 Determine Whether The Series Converges Or Diverges Youtube



Www3 Nd Edu Dgalvin1 S08 Exam3 Solns Pdf
Proof If p 1, the series diverges by comparing it with the harmonic series which we already know diverges Now suppose that p>1 The function f(x) = 1=xp is a decreasing function, so to determine the convergence of the series we'll detemine the convergence of the corresponding integral Z 1 1 1 xp = 1 (p 1)xp 1 1 1 = 0 1 p 1 Determining convergence (or divergence) of a sequence Convergence means that the infinite limit exists If we say that a sequence converges, it means that the limit of the sequence exists as n → ∞ n\to\infty n → ∞ If the limit of the sequence as n → ∞ n\to\infty n → ∞ does not exist, we say that the sequence divergesThe sequence is for n ∈ ℕ divergent all right If we take the quotiënt of the functions f (n)=2^n and g (n)=n², and than use L'Hôpital's rule two times, we arrive at lim n → ∞ (ln (2))²^ (n1)=∞ L'Hôpital's rule Also let f (n)/g (n)=log f (n)/log g (n)=log 2^n/log n²=n*log 2/2 log n=



Question Video Using The Ratio Test To Determine Convergence Nagwa



Teachingcenter Ufl Edu Files Exam 2 Review Problems Mac 2312 Pdf



0 件のコメント:
コメントを投稿