Let f N → Y be a function defined as f (x) = 4 x 3 where Y = {y ∈ N y = 4 x 3} for some x ∈ N such that f is invertible then its inverse is Medium View solutionIe logb(x) = k ⇔ blogbx = bk = x Graphically, we can see that they are inverses because the functions reflect about the line y = x Consider f(x) = log2(x) (in blue) and g(x) = 2x (in red), whose graphs are given below Notice that the domain of y = bx is the entire real line, which is now the range of y = logbx In mathematics, a function(eg f), is said to be an inverse of another(eg g), if given the output of g returns the input value given to f Additionally, this must hold true for every element in the domain codomain(range) of g Eg assuming x and y are constants if g(x) = y and f(y) = x then the function f is said to be an inverse of the function g
2
Inverse function of y e x
Inverse function of y e x- The function has an inverse function because it is onetoone, but its inverse can't be written in closed form Can you give any context?Find a formula for the inverse of the function 1 f(x)=1?(23x) 2f(x)=(4x1)/(2x3) 3f(x)=e 2x1 4y=ln(x3) 5y=(e x)/12e x Expert Answer Who are the experts?
Functions And Their Inverses Worked Examples
To ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW The inverse of the function `f(x)=(e^xe^(x))/(e^xe^(x))2` is given byThe base 10 logarithm function Background Every positive number, y, can be expressed as 10 raised to some power, xThis relationship is described by the equation y = 10 x, and described by this graph For example the number 16 can be expressed as 10 12This is the black dot in the graphThe range of y is To find the inverse function, let's rearrange the avove equation, Therefore, the inverse function is, The denominator cannot be zero at anytime Domain is the range of previous
Finding the Inverse of an Exponential Function I will go over three examples in this tutorial showing how to determine algebraically the inverse of an exponential function But before you take a look at the worked examples, I suggest that you review the suggested steps below first in order to have a good grasp of the general procedureSo, an inverse to a function is found by switching the y and x terms in an equation Your function would be y=e^x Switching the x and y gives you x=e^y That doesn't say anything particularly intuitive So how do you bring the y term down from thFree functions inverse calculator find functions inverse stepbystep This website uses cookies to ensure you get the best experience By using this
Question Find The Formula For The Inverse Of The Function Y= E^x/1 7e^x F^1(x)= This problem has been solved!To find the inverse function of {eq}\displaystyle f(x)=e^{x4} {/eq} we need first the domain and range of the onetoone function The exponential function is oneto Here we are given the function Steps to find inverse are Step 1 First replace f (x) by y Step 2 Replace every x with a y and replace every y with an x Step 3 Solve the equation from step 2 for y Taking log on both sides
2
The Inverse Function X Y The Solid Line Corresponds To The Download Scientific Diagram
Figure 29 A graph of the function y = e x along with its inverse, y = \ln (x), where both functions are viewed using the input variable x In particular, we observe that mA0 = 1 mA and mB0 = 1 mB This is not a coincidence, but in fact holds for any curve y = f (x) and its inverse, provided the inverse existsL Linty Fresh Junior Member Joined Messages 58 #5 Thanks So am I barking up the wrong tree by taking the natural log of both sides?More generally, an exponential function is a function of the form f ( x ) = a b x , {\displaystyle f (x)=ab^ {x},} where b is a positive real number, and the argument x occurs as an exponent For real numbers c and d, a function of the form f ( x ) = a b c x d {\displaystyle f (x)=ab^ {cxd}} is also an exponential function, since it can be
How To Find The Inverse Function In F X X Ln X Quora
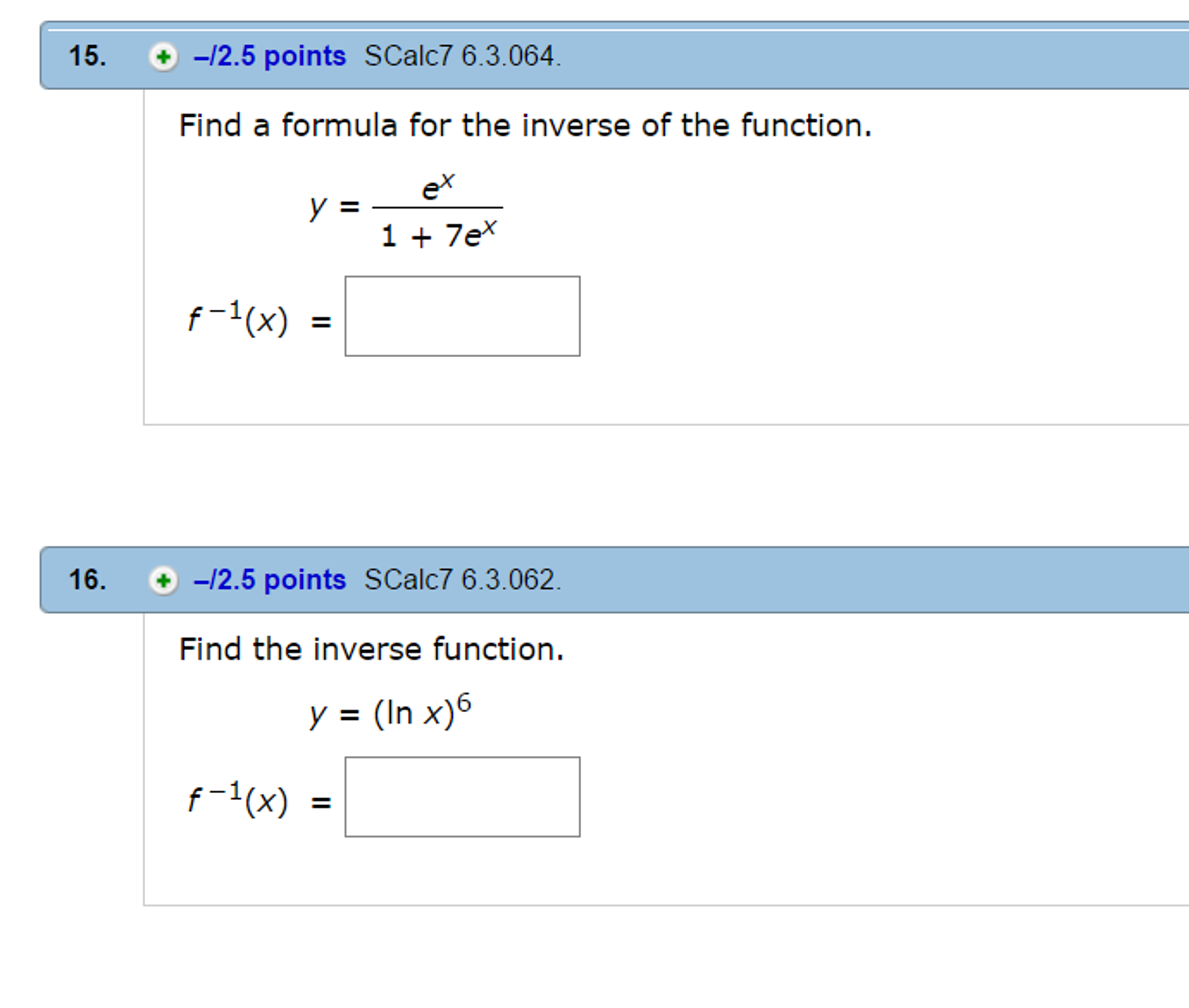
Find A Formula For The Inverse Of The Function Y Chegg Com
As Chris and Isaac noted, you don't actually need to invert the function But in case you want to do that anyway, the way to do it is to view y = e x − 2 e − x as a quadratic equation in e x namely multiply everything by e x to getThe Inverse of Natural Logarithmic Function Suppose we have a logarithmic function {eq}y=\ln(g(x)) {/eq} and we need its inverse formula, then replace the You can use the Lambert W function to solve it symbolically y = xex gives x = W(y) You may run solve (x*exp (x)y,x) on SymPy Live as an alternative to SageMath As indicated in the comments and in another answer, there is a special function that has been defined which can serve as an inverse here
Ex 1 3 6 Show F X X X 2 Is One One Find Inverse Of F
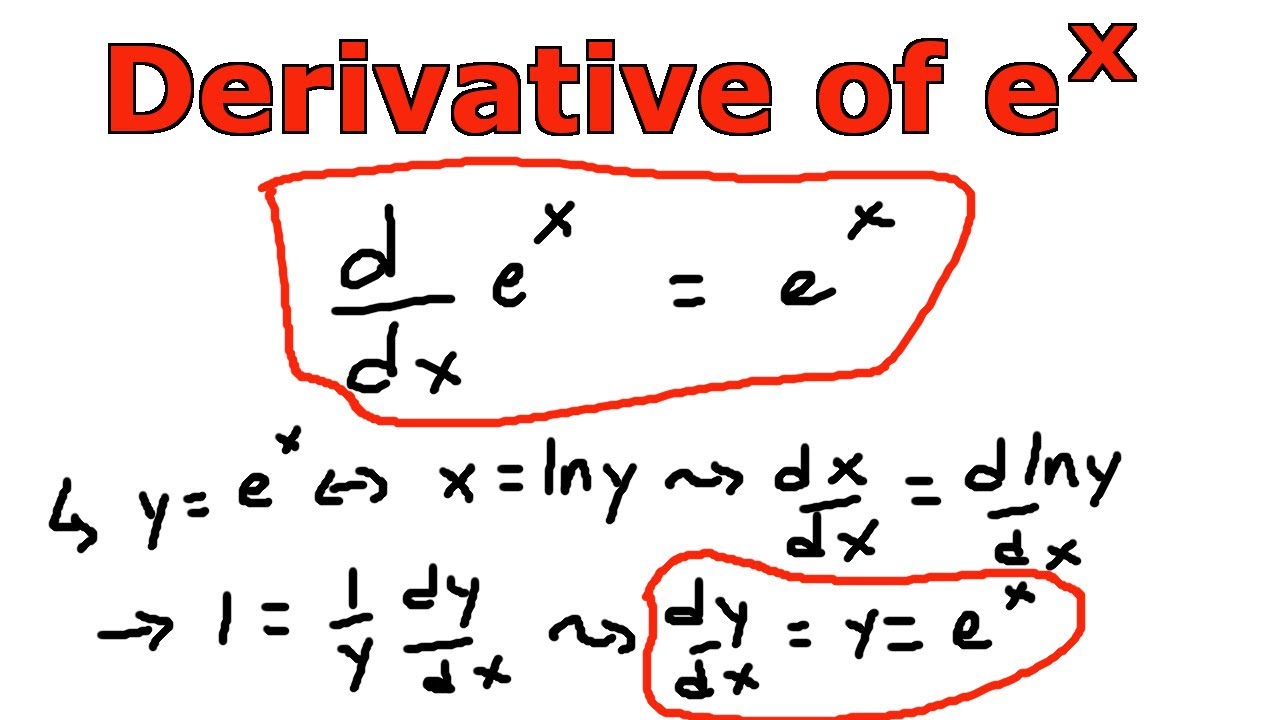
Derivative Of E X Using The Inverse Function Method Youtube
1To nd the inverse of f(x) = ex2 set y= ex2 and then swap inputs and outputs so that x= ey2 Take the natural logarithm of both sides lnx= y2 and solve for yby taking square roots of both sides p lnx= y So one inverse function is f 1(x) = p lnx 2To nd the inverse of y= ex2 5 we swap letters so that x= ey2=5, take natural logs of both sidesFunctions f and g are inverses if f(g(x))=x=g(f(x)) For every pair of such functions, the derivatives f' and g' have a special relationship Learn about this relationship and see how it applies to 𝑒ˣ and ln(x) (which are inverse functions!)In Figure 263, we are reminded that since the natural exponential function has the property that its derivative is itself, the slope of the tangent to \(y = e^x\) is equal to the height of the curve at that point For instance, at the point \(A = (\ln(05), 05)\text{,}\) the slope of the tangent line is \(m_A = 05\text{,}\) and at \(B
Solution Express The Inverse Function Of Y E 4x 5 In The Form Y A Ln X B We Are Looking For The Coefficients A And B I Have Come Up With Y 1 4 Ln X 5
How Do You Find The Inverse Of F X E X Socratic
Inverse Function F X E X 6 Youtube For more information and source, see on this link https//wwwyoutubecom/watch?v=XQ4d2lWDGWEVCE Maths Methods Inverse functions Finding inverse functions one to one 6 • Let fa,∞)→R,where f(x)=x2−6x • If a is the smallest real number such that f has an inverse function, #nd the value of a and the inverse f −1(x) • This is a quadratic function (many to one)I Well just set ax b = y and solve for x I We get x= ( y b)=a So = h( ) = ( I I The inverse function of g(x) = ex is x = h(y) = ln(y) I If gis monotonically increasing, so
What Is The Graph Of E X Quora
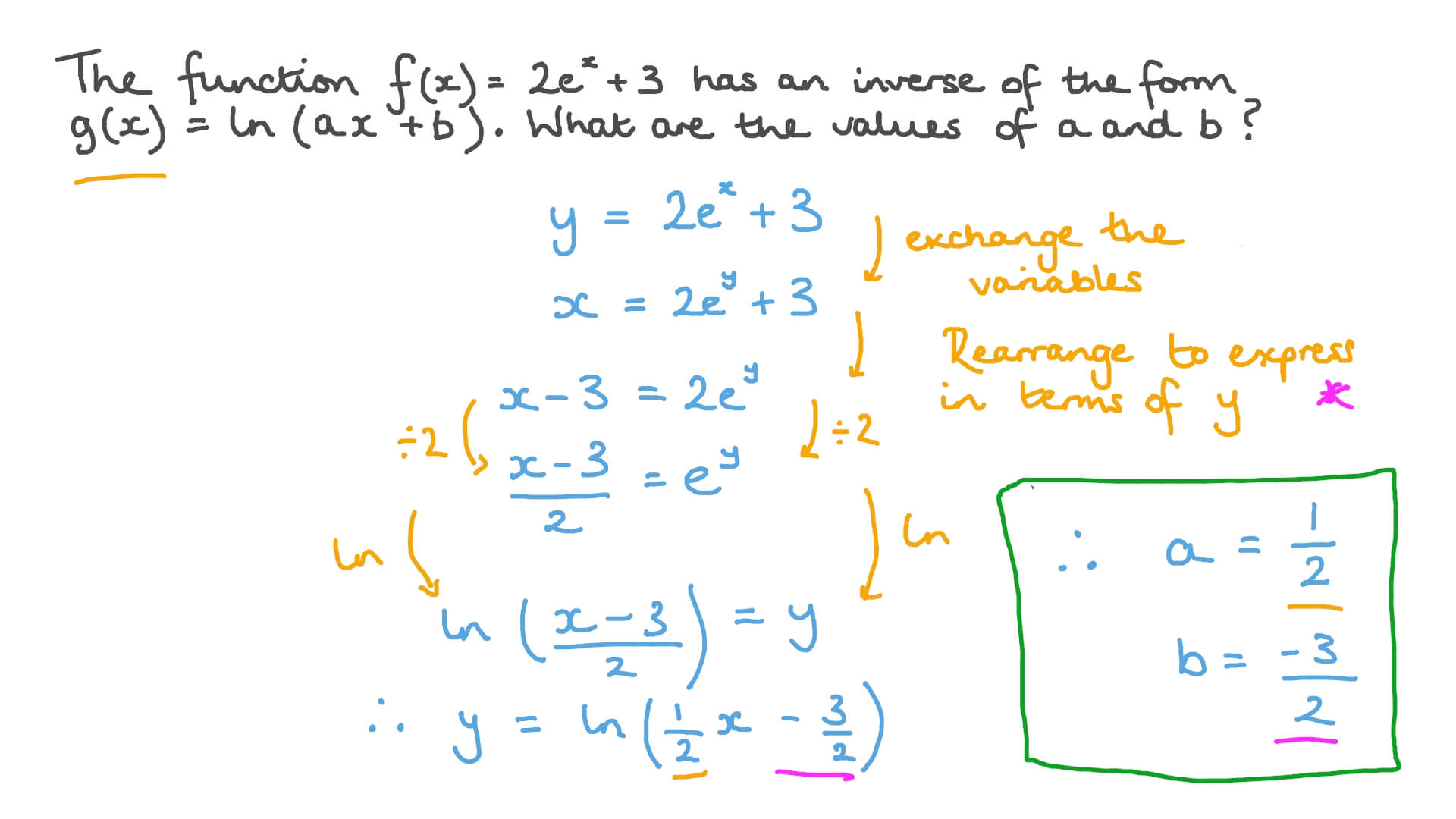
Question Video Finding The Inverse Of A Function Containing An Exponential Function Nagwa
An inverse function h (also known as g 1) such that g(x) = y if and only if x = h(y) For all x 2I I If I tell you that g(x) = ax b, then what is the inverse function? 619 You can't in any reasonably simple way There aren't any standard functions in the book to write the answer with If you want to find say f^ (1) (3) you just have to use numerical methods to get an approximationLatexy=e^x/latex is the only function with this property A Model For Proportional Change The exponential function is used to model a relationship in which a constant change in the independent variable gives the same proportional change (i e, percentage increase or decrease) in the dependent variable
Y 10 X Y Log 10 X Y X The Log 10 X Pronounced Log Base 10 Is Called The Inverse Function Of Y 10 X The Inverse Function Is Always A Reflection Ppt Download
Lecture 3 The Natural Exponential Function F X Exp X E X Y Exp X If And Only If X Ln Y Pdf Free Download
Click here👆to get an answer to your question ️ The inverse of the function f (x) = e^xe^ x/e^xe^ x 2 is given by Join / Login > 12th > Maths > Relations and Functions > Invertible Functions > The inverse of the function maths The inverse of the function Then, y = e x − e − x e x − e − x A mathematical function (usually denoted as f(x)) can be thought of as a formula that will give you a value for y if you specify a value for xThe inverse of a function f(x) (which is written as f1 (x))is essentially the reverse put in your y value, and you'll get your initial x value back Finding the inverse of a function may sound like a complex process, but for simpleRewrite the equation as e y = x e y = x Take the natural logarithm of both sides of the equation to remove the variable from the exponent Use logarithm rules to move y y out of the exponent The natural logarithm of e e is 1 1 Multiply y y by 1 1 Solve for y y and replace with f −1 (x) f 1 ( x)
Graphs E X And Ln X Geogebra
Exponential Function Wikipedia
# log a^n = nlog a# #ln x= y1# #ln x 1 = y# Step 3 Change #y# to #f^(1)(x) # #f^(1Reciprocal function y = e x;Find the inverse function, its domain and range, of the function given by f(x) = e x3 Solution to example 1 Note that the given function is a an exponential function with domain (∞ , ∞) and range (0, ∞) We first write the function as an equation as follows y = e x3;
Exponentials And Logarithms Maths A Level Revision
2 6 Derivatives Of Inverse Functions Mathematics Libretexts
93 The exponential function In this section, we define what is arguably the single most important function in all of mathematics We have already noted that the function lnx is injective, and therefore it has an inverse Definition 931 The inverse function of ln(x) is y = exp(x), called the natural exponential function Given #y= e^(x1)# (this is a onetoone function) Step 1 Switch #x# for #y# and #y# for #x# like this #color(red) x= e^(color(blue)(y)1)# Step 2 Begin to solve for #y# Take #ln# of both side #ln(x) = lne^(y1)# Use the properties of log #ln e= 1#;For functions of more than one variable, the theorem states that if F is a continuously differentiable function from an open set of into , and the total derivative is invertible at a point p (ie, the Jacobian determinant of F at p is nonzero), then F is invertible near p an inverse function to F is defined on some neighborhood of = ()
Derivatives Of Inverse Functions Video Khan Academy
Find The Inverse Of The Function Y E 2x 1 5 X Chegg Com
Note that the inverse will not be a function in this case, since the original function doesn't pass the horizontal line test (thus the inverse will not pass the vertical line test) (Note that some teachers will have you solve for \(x\) and then switch the \(x\) and the \(y\) at the end to get the inverse functionFinding the Inverse of a Logarithmic Function Finding the inverse of a log function is as easy as following the suggested steps below You will realize later after seeing some examples that most of the work boils down to solving an equation The key steps involved include isolating the log expression and then rewriting the Inverse of Logarithmic Function Read More »Experts are tested by Chegg as specialists in their subject area We review their content and use your feedback to
Find A Formula For The Inverse Of The Function Y Chegg Com
Find The Derivative Of E X Sin X With Respect To X
Inverse function function that "reverses" another function if the function f applied to an input x gives a result of y, then applying its inverse function g to y gives the result x, and vice versa ie, f (x) = y if and only if g (y) = x An inverse function is a concept of mathematicsNatural log (inverse xfunction of e ) Recall that aΔx 1 M(a) = lim − Δx→0 Δx is the dvalue for which ax = M(a)ax, the value of the derivative of a x when dx x = 0, and the slope of the graph of y = ax at x = 0 To understand M (a) better, we study the natural log function ln(x), which is the inverse of the function ex This function is e x ( 2 y − 1) = − y e x = − y 2 y − 1 / l n x = ln y 1 − 2 y So, for the inverse function we have f ¯ ( x) = ln x 1 − 2 x
Finding The Inverse Of Ln X Video Lesson Transcript Study Com
Inverse Of Y Xe X Mathematics Stack Exchange
The figure given below describes a function and its inverse This function is represented as f (x) and takes some input values and gives an output The inverse of this function is denoted by f1(x) For example, let's say f (x) = 2x It doubles the number which is given as input, its inverse should make them half to get back the input f1(x) = The inverse function is lnx By definition, y=f^(1)(x)ifff(y)=x iffe^y=x iffy=lnxProof of the sinh 1 formula Using the procedure for nding inverse functions, set y = e x 2 Solving for x, we get 2y = ex e x 0 = ex 2y e x 0 = e x e2x 2yex 1 = e x ex 2 2yex 1 e xnever equals zero, but we can use the quadratic formula to solve for e in the second factor ex = 2y p (2y)2 4 1 ( 1) 2 = 2y p 4y2 4 2 Since ex cannot be negative, we can ignore the \ " answer = y p y2 1
Find A Formula For The Inverse Of The Function Y Chegg Com
If Y E X Sin 2 X Sin X X Find Dy Dx With Video Teachoo
The derivatives of the inverse trigonometric functions are as follows d dxarccot(x) = − 1 1 x2 d dxarcsec(x) = 1 x2√1 − x − 2 d dxarccsc(x) = − 1 x2√1 − x − 2 Note that some of these functions are not valid for a range of x which would end up making the function undefinedState the domain and range of `y=e^(x)` and then find its inverse function The parent function is `y=e^x` which has a domain of all real numbers and a range y>0 The transformed functionThe following Key Ideas give the derivatives and integrals relating to the inverse hyperbolic functions In Key Idea 744, both the inverse hyperbolic and logarithmic function representations of the antiderivative are given, based on Key Idea 742 Again, these latter functions are often more useful than the former
Exponential Function Wikipedia
The Logarithmic Function Y Ln X Is The Inverse Of Gauthmath
Here we want to find inverse of e x Let, y=e x Then swap the variables x and y in y=e x and solve it for x Swapping the variables in y=e x we get, x=e y Then taking logarithm on both sides of x=e y we have, ln(x)=ln(e y) =y (since, by logarithmic property ln(e x)=x) So, y=ln(x) Hence, ln(x) is the inverse function of y=e x Answer) ln(x)In this video I go over the natural exponential function y = e^x and define it strictly from looking at the inverse of the natural logarithmic function y = lInverse function y = log e x or y = ln(x) slope itself, y = exp(x) or y = e x The exponential function is a power function having a base of e This function takes the number x and uses it as the exponent of e For values of 0, 1, and 2, the values of the function are 1, e or about 2718, and e² or about 7
Inverse Functions
6 Derivative Of The Exponential Function
See the answer Show transcribedFind the Inverse y=4^x y = 4x y = 4 x Interchange the variables x = 4y x = 4 y Solve for y y Tap for more steps Rewrite the equation as 4 y = x 4 y = x 4 y = x 4 y = x Take the natural logarithm of both sides of the equation to remove the variable from the exponentWhy do you want to do this?
Find The Inverse Function Y E X 1 2e X F 1 X Chegg Com
Untitled Document
Search Q Inverse Of Ln Tbm Isch
6 Derivative Of The Exponential Function
2
1
1
The Inverse Of The Function F X E X E X E X E X 2 Is
Lesson 45
Find An Equation Of The Tangent Line To The Curve Y Chegg Com
Exponential Function Definition By Amit Kumar Issuu
Copyright C Cengage Learning All Rights Reserved Ppt Video Online Download
The Logarithmic Function Y Ln X Is The Inverse Of Gauthmath
5 Consider The Function Y E Then A The Function Chegg Com
How Do You Solve Lnx 7 Socratic
Find A Formula For The Inverse Of The Function Y Chegg Com
Example 5 Use Inverse Properties Simplify The Expression
What Is The Inverse Of E X Quora
Inverse Functions Recap Of Inverse Of A Function Inverse Functions With E X And Ln X Ppt Download
Inverse Functions Recap Of Inverse Of A Function Inverse Functions With E X And Ln X Ppt Download
Mathematics Ii Tranquileducation
Solution How Do I Find The Inverse Function Of F X Ln E X 2 I Started It Like This F X Y Lt Gt Y Ln E X 2 Lt Gt E Y E X 2 Lt Gt E 2y E X 2 Lt Gt E X
12x1 T05 01 Inverse Functions 10
1
Natural Exponential Function And Natural Logarithmic Function Mathbitsnotebook Ccss Math
Inverse Of Y Xe X Mathematics Stack Exchange
Natural Exponential Function Y E X Youtube
The Real Number E Boundless Algebra
Ppt The Function E X And Its Inverse Lnx Powerpoint Presentation Free Download Id
2
Inverse Cubic And Exponential Functions Geogebra
Exponential And Logarithmic Functions The Natural Log
7 Inverse Functions Inverse Functions 7 3 The
Functions Inverses Ppt Download
What Is The Graph Of E X Quora
Example 2 Verify That Y E 3x Is A Solution Of Y Y 6y 0
Find The Largest Intervals A Y B Where The Function Y Xe X Has An Inverse Function X X Y Differentiable On A B Study Com
Functions And Their Inverses Worked Examples
2
Differentiating Exponential And Logarithmic Functions
Inverse Hyperbolic Functions
Ex Find An Inverse Function From A Table Youtube
Logarithmic Functions
Natural Logarithms
What Is The Inverse Function For Math F X E X Math Quora
2
Inverse Function For F X 2 E X 2 Youtube
Ex 2 Find The Inverse Of A Function Youtube
6 Derivative Of The Exponential Function
Inverse Functions Part 1
Inverse Function F X Ln X 10 Youtube
Chapter 28 Models Of Evolution Figure 28 5 The Inverse Of The Exponential Function Y Ex Is The Natural Logarithm X Log Y
Parent Functions Types Properties Examples
2
The Real Number E Boundless Algebra
State The Domain And Range Of Y E X Then Find The Inverse Equation Of The Function Enotes Com
Inverse Function F X E X 6 Youtube
Finding Inverse Functions Quadratic Example 2 Video Khan Academy
7 Inverse Functions Inverse Functions 7 3 The
3 Natural Exponential Function Exponential Function Logarithm
2
E Mathematical Constant Wikipedia
How Do You Convert From Exponential Form To Natural Logarithmic Form Printable Summary Virtual Nerd
2
Natural Logarithms
Find The Inverse Function Y E Quberoot X Select Chegg Com
Answer In Calculus For Neha
What Is The Inverse Of E X Quora
Differentiation Of The Exponential Function E X And Natural Logarithms Lnx Exponential Function E X Ppt Download
Inverse Of Derivative Function The Integral
1
Natural Exponential Function And Natural Logarithmic Function Mathbitsnotebook Ccss Math
Natural Exponential Function And Natural Logarithmic Function Mathbitsnotebook Ccss Math
Exponential Function Wikipedia
Find The Minimum Distance Between The Curves Y E X And Y Ln X Study Com
By Rafal Paola Mujahid Y E X Exponential Function Logarithm Function Is The Inverse Ex1 Y Log 4 Xy 4 X Therefore Y E X Y Log E X Ppt Download
0 件のコメント:
コメントを投稿